- Graphisoft Community (INT)
- :
- Developer Hub
- :
- GDL
- :
- Re: Find X and Y of where line intersects with cha...
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Pin this post for me
- Bookmark
- Subscribe to Topic
- Mute
- Printer Friendly Page
Find X and Y of where line intersects with chamfer / fillet
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Report Inappropriate Content
2022-08-02 05:15 PM
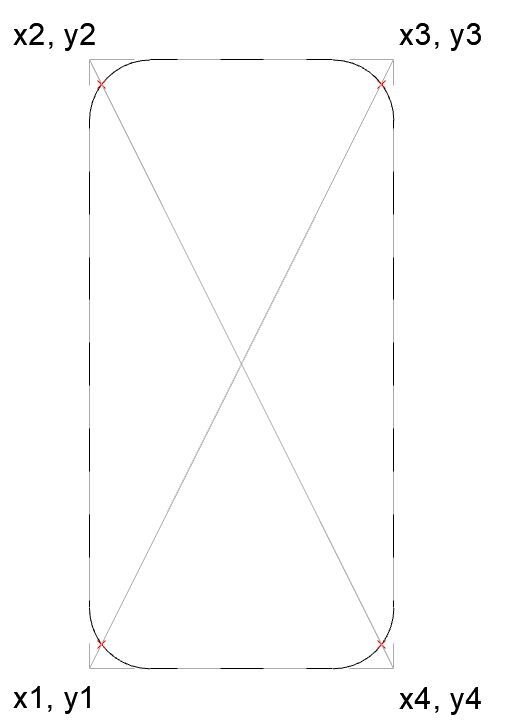
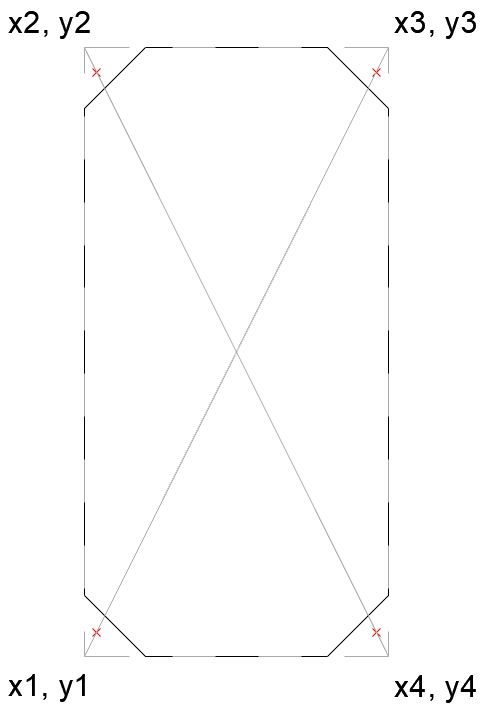
I have a box where any of the corners can be filleted or chamfer... or just a plain right angle.
My goal is for the diagonal lines to come to a stop when they reach the chamfer or fillet and not extend past to the corner. Hopefully the images attached demonstrate this.
Is there anything similar to a cutpoly for 2D? I haven't been able to find a formula to figure out how to calculate the x and y of the points.
Any help would be very much appreciated,
Thanks
Windows 10
- Labels:
-
Library (GDL)
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Report Inappropriate Content
2022-08-03 03:41 AM - edited 2022-08-03 03:43 AM
You know the coordinates of the two ends of your diagonal line.
From those two ends, you can work out the equation for the line that runs between the two.
You know where your chamfer corners are.
From your chamfer corners, you can work out the equation for the line that runs between the two.
You now have two equations. Well, four if you are working your x and y separately... Anyway, now with your two equations, you can use an IF statement for when they equal each other. When they do, x (diagonal) = x (chamfer) and y (diagonal) = y (chamfer)
The fillet will be a similar process using x^2 + y^2 = r together with your circles centre offset. You will get two intersection points with this though so you will need to eliminate them depending on which corner you are solving for.
Ling.
| AC22-28 AUS 3110 | Help Those Help You - Add a Signature |
| Self-taught, bend it till it breaks | Creating a Thread |
| Win11 | i9 10850K | 64GB | RX6600 | Win11 | R5 2600 | 16GB | GTX1660 |
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Report Inappropriate Content
2022-08-23 09:39 AM
There is no language support for this, but we have
- BasicGeometry / INTERSECT_LINE_POLYGON_2D in the Archicad Library, or
- PolyOperations / ClipPolyline for very generic cases.
Software Engineer, Library
Graphisoft SE, Budapest
